All that can go wrong with recirculation

[Art Carlson]

Whatever the sins of cusp confinement, it seems popular to absolve them by invoking recirculation. I see this concept to be just as weak as whiffle ball confinement. Remember that the particles are coming out from a very small spot and/or in a very narrow cone. Recirculation requires that this special flux tube and/or velocity distribution match up with the special flux tube and/or velocity distribution that would allow the particles to come back in another place. If these special conditions are met, then anything that jostles them along the way will destroy the special conditions and the particles will be trapped out in the cold.

But first, do we expect the field lines of one cusp to map to the field line of another cusp? As a matter of fact, we do. There is not much that is easy to understand and express about the 3-d magnetic geometry of a polywell, but one of those things is that the field lines passing through the middles of the faces, sides, and corners of the cube are straight as an arrow. They penetrate all the way to the zero-field middle of the cube (which makes them cusps) where they intersect, and they extend all the way to infinity on the outside, where (mathematically) they also intersect. Oops. Is that what we wanted? A field line near one cusp will return to a point near another cusp, but it will take the long way around to get there, usually running into the containment vessel before it has a chance to recirculate.

And while we're talking about running into things, we all agree we want to push to the highest field technically possible, both for good confinement and high power density. This implies a some hefty magnet coils, to provide conductor cross section, to provide cooling, and to provide mechanical support against magnetic forces, and the feeds for these coils must be equally hefty for the same reasons. Why shouldn't I expect several percent of the plasma fan leaving a cusp to run into these feeds?

But we're not finished yet. Suppose we've got a flux tube that leaves one cusp and enters another without hitting anything inbetween. Will the plasma really follow it? Any electric fields - and they are postulated by the concept to be large - will shift the plasma off the flux tube through the EXB (E cross B) drift. Isn't it likely to land next to the tiny hole it was aiming for instead of in it? There are also the grad B and curvature drifts. Again, the magnetic field gradients and curvature will definitely be large. These are especially evil because they act in opposite directions for positively and negatively charge particles, leading to charge separation and a powerful EXB drift again. This is where the "bad curvature" of the magnetic field raises its head, the one that proponents are so proud that the polywell, in contrast to the evil tokamak, does not have. Well, the curvature inside the coil system is all good, but the curvature outside is all bad, and it will wreak havoc with your recirculation.

Rick Nebel is going to come back and explain that the idea of recirculation along flux loops is passe, that the current thinking is that most electrons will be turned around by the electric field between the magrid and the housing and be recirculated through the same cusp they came out of. If that happens, it will quantitatively reduce the effects I list, but not eliminate them. All these things will still be going on for the duration of the particles' excursion out of the cusp. But in fact there is no reason to expect such a local recirculation. The charge on the coils, if it is not hidden entirely by a Debye sheath, will result in an electric field at right angles to the magnetic field. There's that EXB drift again. In any self-respecting plasma, the free and easy motion of charges parallel to B will (nearly) eliminate the E field in that direction. There is also a contradiction is the idea of using the radial electric field to confine electrons *and* ions. If the field recycles electrons at some location, then it will spit out ions there, and vice versa.

This should do for a start. I believe the true believers talk about getting recirculation factors of 1000 or 10,000 (because that is what they need to compensate for the poor cusp confinement, which I argue is even poorer than they think). I can't imagine getting more than a factor of 10 (although this is hard to quantify), and I suspect you will actually get much less than that.

[TallDave]

Whatever the sins of cusp confinement, it seems popular to absolve them by invoking recirculation. I see this concept to be just as weak as whiffle ball confinement. Remember that the particles are coming out from a very small spot and/or in a very narrow cone. Recirculation requires that this special flux tube and/or velocity distribution match up with the special flux tube and/or velocity distribution that would allow the particles to come back in another place. If these special conditions are met, then anything that jostles them along the way will destroy the special conditions and the particles will be trapped out in the cold.

I think this is a function of the shape of the fields. They're apparently friendlier on the outside, sort of a like a bunch of funnels stuck on the outside of a wiffle ball.

A field line near one cusp will return to a point near another cusp, but it will take the long way around to get there, usually running into the containment vessel before it has a chance to recirculate.

A lot of us thought so too, but it turns out the fields actually wrap pretty tightly because of the way four coils interact. There's a picture of this counter-intuitive result somewhere. Also, notice that WB-7 in the pic at EMC is very close to the wall.

Why shouldn't I expect several percent of the plasma fan leaving a cusp to run into these feeds?

I think Bussard calculated the acceptable intercept area and found the supports were acceptable.

Well, the curvature inside the coil system is all good, but the curvature outside is all bad, and it will wreak havoc with your recirculation.

I don't see why this would be so. The magetic field should always be convex to where the electron wants to go because it always wants to get to the Magrid.

This should do for a start. I believe the true believers talk about getting recirculation factors of 1000 or 10,000 (because that is what they need to compensate for the poor cusp confinement, which I argue is even poorer than they think). I can't imagine getting more than a factor of 10

Unless we don't believe them, they have already seen this experimentally.

Out of curiosity, how does one measure this? is it simply a question of well depth versus drive?

[rcain]

This should do for a start. I believe the true believers talk about getting recirculation factors of 1000 or 10,000 (because that is what they need to compensate for the poor cusp confinement, which I argue is even poorer than they think). I can't imagine getting more than a factor of 10

Unless we don't believe them, they have already seen this experimentally.

Out of curiosity, how does one measure this? is it simply a question of well depth versus drive?

how about Laser-induced fluorescence (LIF) ( eg: http://www.telegrid.enea.it/Conferenze/ ... /11_08.PDF ) ?

[93143]

Why shouldn't I expect several percent of the plasma fan leaving a cusp to run into these feeds?

I believe the feeds on WB-6 were ceramic coated. Dielectric. That way, they rapidly accumulate a negative charge, and the electrons start to avoid them. (I could be misinterpreting.)

Also, I remember hearing something about what TallDave said regarding the electron flux in the vicinity of the supports being predicted to be small.

But we're not finished yet. Suppose we've got a flux tube that leaves one cusp and enters another without hitting anything inbetween. Will the plasma really follow it? Any electric fields - and they are postulated by the concept to be large - will shift the plasma off the flux tube through the EXB (E cross B) drift. Isn't it likely to land next to the tiny hole it was aiming for instead of in it?

That may be a good point. I have some half-baked ideas, but nothing good. A thought - the electron, unless it hits the cusp dead center, is going to be gyrating across significant transverse B field gradients as it traverses a steep axial B field gradient. It could wind up almost anywhere, and most of the volume of the exterior B field leads back in another cusp - it's not a tiny hole when you're coming in from the outside.

Still, that's a weak argument. I'm not an expert on plasma yet...

Well, the curvature inside the coil system is all good, but the curvature outside is all bad, and it will wreak havoc with your recirculation.

I think I see what you mean, but the electron density out there is very low, so it shouldn't matter much. The magnetic field has a fair bit of authority.

Wait, isn't the field convex out there too? As in, convex in the direction of decreasing field? Or is that not what that means?

But in fact there is no reason to expect such a local recirculation. The charge on the coils, if it is not hidden entirely by a Debye sheath, will result in an electric field at right angles to the magnetic field. There's that EXB drift again. In any self-respecting plasma, the free and easy motion of charges parallel to B will (nearly) eliminate the E field in that direction.

But this isn't a self-respecting plasma. It's an excursion of electrons - no ions - at high (and fairly uniform) energy. It can't really be thought of as a conducting plasma; its behaviour is simple and governed by the principle of superposition. The electric field from the electrons causes beam spreading, but it won't cancel the large E field from the magrid, and the powerful B field should help prevent spreading (in a full-size machine, the B field is in the ITER range, and we're talking about electrons here).

Of course, radial beam spreading may cause something of the same problem you postulated regarding the alternate-cusp recirculation, and the magrid could join in - although its field is radial at the exact cusp centerline...

EDIT: Nothing to see here... Apparently I can't address Debye shielding without saying something dumb. You'd never guess I did my M.Sc. in multiphase electrokinetics...

There is also a contradiction is the idea of using the radial electric field to confine electrons *and* ions. If the field recycles electrons at some location, then it will spit out ions there, and vice versa.

The answer to this one lies in the IEC concept and the non-Maxwellian ion energy distribution. If the ions are formed at some nonzero depth into the well with ECR, they should almost never escape outside the magrid. The electric field is what confines them, and it has no cusps.

If we do get a small population of electrons orbiting the coils, they could serve to make the wiffleball start partway down the well, rather than at the top. This could improve the resistance of the ion confinement to partial thermalization, if the ECR is tuned to the edge of the wiffleball. Just a thought...

This should do for a start. I believe the true believers talk about getting recirculation factors of 1000 or 10,000 (because that is what they need to compensate for the poor cusp confinement, which I argue is even poorer than they think). I can't imagine getting more than a factor of 10 (although this is hard to quantify), and I suspect you will actually get much less than that.

I suspect we'll find out for sure in a few months. Dr. Nebel probably already knows, and he seems happy. Experimentum solum certificat in talibus...

[Roger]

There's a picture of this counter-intuitive result somewhere.

Something like this ?



From here:

http://www.youtube.com/watch?v=jmp1cg3-WDY

[TallDave]

Roger,

Actually, I was thinking of a pic that showed the field gradients with colors.

With one or two coils, you got a field that spread out quite a bit. With four, the field tended to wrap.

[Roger]

Sounds like Indreks

[MSimon]

But we're not finished yet. Suppose we've got a flux tube that leaves one cusp and enters another without hitting anything inbetween. Will the plasma really follow it? Any electric fields - and they are postulated by the concept to be large - will shift the plasma off the flux tube through the EXB (E cross B) drift. Isn't it likely to land next to the tiny hole it was aiming for instead of in it?

I think recirculation is a misleading term. Oscillation is a better description.

There are a lot of subtle points and the current terms of the art are not always best.

[tonybarry]

A lot of us thought so too, but it turns out the fields actually wrap pretty tightly because of the way four coils interact. There's a picture of this counter-intuitive result somewhere. Also, notice that WB-7 in the pic at EMC is very close to the wall.

Hello Dave,
Your image is here:-



Please note this represents a 2D slice through the magrid equator; and perhaps more importantly represents the magnetic field only. The resultant field when electrons are added will be different. The colour scale is an automatically added effect and vary between images; the contours are correct in both images and are at 2, 1, 0.5, 0.2, 0.1Tesla respectively.

EDIT ADD:-
The "counter intuitive" observation can be explained in casual terms by considering that the arrangement of the coils "kills" the far-field effects of the magnetic field induced by the current flowing in the coils. Thus, right near to the skin of the coils you have a large magnetic field, essentially unchanged by items around it; the further out you go, the more the other coils' fields destructively interfere with the magnetic field of our original coil. End result (and the one that surprised me initially) - the magnetic field does not balloon out the back of the MaGrid as one might expect. Hence the nearness to the outer casing of the WB-7 pic on the EMC2 website.

Regards,
Tony Barry
[/img]

[Art Carlson]

A field line near one cusp will return to a point near another cusp, but it will take the long way around to get there, usually running into the containment vessel before it has a chance to recirculate.

A lot of us thought so too, but it turns out the fields actually wrap pretty tightly because of the way four coils interact. There's a picture of this counter-intuitive result somewhere. Also, notice that WB-7 in the pic at EMC is very close to the wall.

We may need to get down to brass tacks here. What we know for sure is that the field line through the center of the cusp goes straight to the wall. The quantitative question is something like the maximum radial excursion of a field line which in the cusp is about one gyroradius off-center. I don't see how to read that from the illustrations reproduced in this thread. I tried to estimate this distance and got a value several times greater than the device radius, but I don't trust it yet. Can somebody plug this question into his code?

Well, the curvature inside the coil system is all good, but the curvature outside is all bad, and it will wreak havoc with your recirculation.

I don't see why this would be so. The magetic field should always be convex to where the electron wants to go because it always wants to get to the Magrid.

Even outside the coils you will have a quasineutral plasma. This effect requires the electrons and ions to work together (and against us!). Also don't forget that electrons don't "want" to go to positively charged objects. What they want to do in an electric field is to drift perpendicular to it and the magnetic field. But you're half right. The curvature toward the walls is bad, but the curvature toward the coils is good.

This should do for a start. I believe the true believers talk about getting recirculation factors of 1000 or 10,000 (because that is what they need to compensate for the poor cusp confinement, which I argue is even poorer than they think). I can't imagine getting more than a factor of 10

Unless we don't believe them, they have already seen this experimentally.

Can you cite anyone (Bussard or Nebel) actually saying this? I've heard a lot of hearsay on this forum that didn't turn out to be accurate. And after having looked more closely at the way Bussard works (see the thread "Where's the beef?"), I am inclined not even to believe conclusions he states directly unless I can see the data myself.

[TallDave]

Thanks tonybarry.

Even outside the coils you will have a quasineutral plasma.

Huh? That's not my understanding. I was under the impression the ions generally don't make it outside the device. Remember when Nebel said the Larmor radius was essentially infinite for the ions because they almost never see the B field?

Can you cite anyone (Bussard or Nebel) actually saying this?

I think Nebel said it just the other day in one of these threads. IIRC, he stated it the difference was "obvious" or words to that effect.

Here we go:

1. There were some questions about how one knows that you have "wiffleball" confinement as opposed to "mirrorlike" confinement. The answer is that there are about 3 orders of magnitude difference in the confinement times between the two modes. That kind of difference is easy to see.

Still not sure how exactly they measure this, but it does seem like it should be hard to miss.

[Indrek]

The field lines would direct the electrons way away from the machine. Recirculation as in making circles out one cusp and in another would not work. Have a look at this image of the field lines:



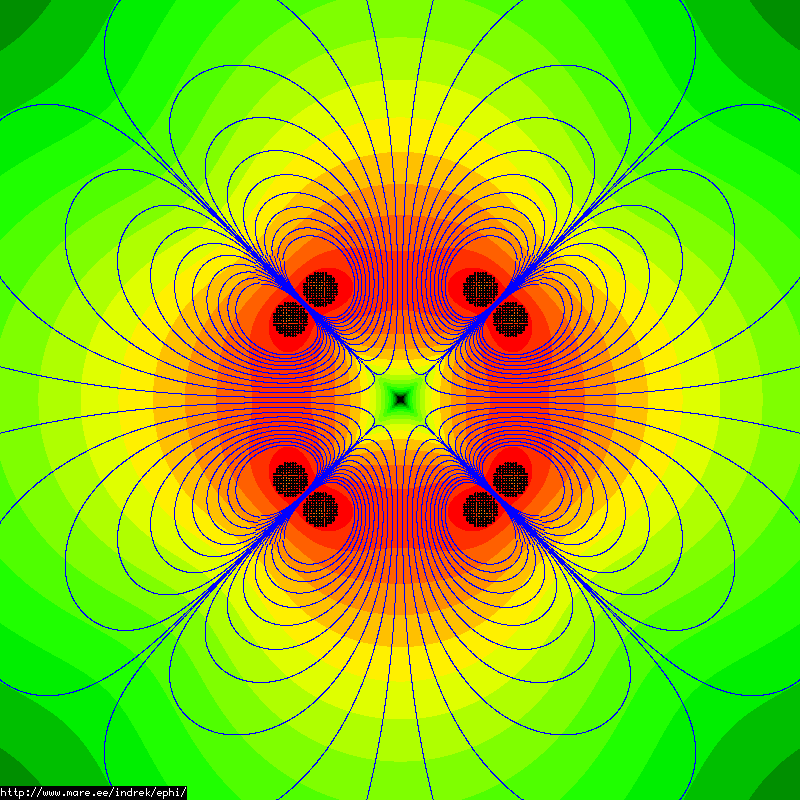
The blue lines are the b-field lines (should be correctly spaced) and the color is bfield magnitude (logarithmic levels). Here radius=0.15m, coil thickness=0.07m, coil spacing=0.01m.

So only back-and-forth would work.

- Indrek

[TallDave]

The field lines would direct the electrons way away from the machine.

They would try, but they have to fight the electrostatic attraction back to the Magrid.

Again, they have WB-7 awfully close to the wall. That implies the electrons hew pretty close to the machine.

[Art Carlson]

Thanks, Indrek. That should give us a useful reference point. If we say that the outer limits of your diagram are the sort of dimensions we are thinking of for the outer box of a reactor, then we can say a field line in the cusp that is off-axis by about 1/30 of the coil radius will just reach the outer wall. In a reactor with dimensions on the order of 1 m, that would be 30 mm. The electron gyroradius at 10 T and 100 keV would be around 0.1 mm. Therefore all the particle (ions will be even worse than electrons) escaping the cusp will definitely intersect the wall. If you can't manage to hold them back electrostaticly, the (recirculation) game is over.

[MSimon]

If you can't manage to hold them back electrostaticly

That is the whole game.