Why is polywell supposed to be better than cusp confinement?

[seedload]

If line cusps have the same throughput as point cusps, yes; but I just posted a plot showing that the point cusps are the major loss factor.

I just wanted to say that it is really cool how much your picture looks like the picture of the WB7 in operation on the EMC2 website. Clearly, most of the junk is going out the point cusps.

I think Art is arguing that in wiffleball mode the line cusps will dominate.

regards

[scareduck]

We are all really waiting for rnebel to provide the data he mentioned in his post on the first page of this thread.

[TallDave]

Even if the line cusps wind up adding a power of R to the predicted losses, we can just make the machines bigger by a power of 5/4, right? That's not that bad...

Bussard also believed a dodec would be 3-5 times better, which might alleviate that.

[kcdodd]

If line cusps have the same throughput as point cusps, yes; but I just posted a plot showing that the point cusps are the major loss factor.

I just wanted to say that it is really cool how much your picture looks like the picture of the WB7 in operation on the EMC2 website. Clearly, most of the junk is going out the point cusps.

I think Art is arguing that in wiffleball mode the line cusps will dominate.

regards

If line cusp loss dominates in WB mode, then logically that means at some point before WB mode is reached the loss through the point cusps will fall lower then loss through line cusps. Which means that line cusps would have to close at a slower rate then point cusps. If line cusps close at the same rate, or higher, as point cusps then point cusps should always dominate. So what would cause line cusps to close slower then point cusps as beta increases?

More over, a funny cusp is just the intersection point of three line cusps. Which begs that even if point cusps close faster then line cusps, does a funny cusp close any faster then a line cusp. If not then you still just have 6 "strong" point cusps and 8 "slightly weaker" funny cusps even assuming line cusps "try" to dominate. And taking into account that funny cusps will almost always be in higher B-field areas than point cusps, they may be just as strong in scaling depending on just how much faster point cusps close compared to funny cusps. Or is my logic wrong?

[tonybarry]

Hello Carter,
I think your logic is sound. Unfortunately when dealing with plasmas, experiment has traditionally guided theory (according to Joe Khachan, the local IEC guy at the Uni. of Sydney). For this reason, we really need experimental measurements of the fields around a working polywell to point us in the right direction. Once we have evidence, we can go forward.

Regards,
Tony Barry

[kcdodd]

I'm just attempting to make an answer to Art Carlson's conceptual objections against polywell in the absence of experimental data. Which seems the only point to the thread.

[MSimon]

I'm just attempting to make an answer to Art Carlson's conceptual objections against polywell in the absence of experimental data. Which seems the only point to the thread.

That is the most certain point on which almost all agree. We need more data. What we have is interesting but not conclusive. Hence WB-7.

Now of course we have no certainty that the efforts will bear fruit, but it is interesting that Rick mentioned at this site that he was interested in selling WB-7s for experimental purposes. I don't think he would be doing that if the results obtained were not useful. However, indications are not data.

[Art Carlson]

I did this plot several months back of individual electron trajectories. As you can see the line cusps receive nearly zero flux. Only one path actually penetrated the line cusp from all the trials, it myst hit the cusp directly or the internal structure of the magnetic field funnels any electrons headed toward the line cusp to a corresponding corner intersection of three of the faces. So these corners seem to receive flux nearly identically to the point cusps of the faces. Bussard knew they were structurally completely different than the point cusps of the faces, but yet they acted almost identically almost as if they were point cusps. I think he called them funny cusps or something. The face point cusps and corner funny cusps were the only gates into or out of the core.

Now, in high beta operation I think he assumed they would have similar relative properties and treated the funny cusp pretty much as the point cusp, knowing they are not the same thing. Now, you can of course argue that at high beta the magnetic structure is not the same so perhaps they do not cause these funny cusps at all. But assuming it did for a second, and assuming the wiffle ball effect at high beta had closed down the gates through the corners and faces, so to speak, you have attained high confinement.

Very impressive, Carter. I am amazed at the tools you guys have, and you seem to know how to use them well enough that you don't bash your thumb too often. I am hard to convince, but this is the type of work that might be able to do it.

1) I think I need some numbers, though. If I understand Bussard (and if he is right), the confinement is much worse at low beta, so it is possible that the line cusps are spewing out their R*rho worth, but the corner cusps are for some reason much much worse in this regime. Can you count up the particles exiting each of the three regions and express that as an effective loss area?

2) Once you are capable of measuring the loss areas, it would be a big step forward to reproduce the consensus results for a bi-conical cusp. You would just need to turn off four of the six coils.

3) It is somewhere between possible and likely that you need to do some work to get into the high beta regime of interest. The easiest way I can think of to simulate that would be to put a superconducting ball into the middle of the configuration and to start the electrons from the surface of this. This may not be as hard as it sounds. I think if you go to circular coils, you can use image coils within the sphere to produce a magnetic configuration with zero normal field on the surface of the sphere.

4) Next, I would like/need to understand what went wrong with my reasoning. Otherwise there may be something important we are missing in this calculation. Could you trace field lines as well as particle trajectories? I would like to see a map from the center of the machine (or the surface of the high beta sphere) to the outside. (Those line cusps just must be there, one way or another!)

There. That's a big shopping list, but it seems to be in the realm of the possible. If we (meaning you, or possible someone else) could carry this out, we might even be able to come to an agreement in the end and make a real contribution to understanding this strange beast.

[seedload]

So what would cause line cusps to close slower then point cusps as beta increases?

Because point cusps close in two dimensions and line cusps close in only one. Assuming a retangular approximation of the cusps, the point cusps shrink in both length and width. The line cusps shrink in only width but not in length. Length is constant.

"Soccer Ball Mode"

[kcdodd]

I posted several plots here: http://andromedaspace.com/fusor_matlab.php. It includes a plot of the magnetic field at the top, and further down the page there is a plot of electrons shot directly at the center of the line cusp. I was very surprised when I did this because variation of a few degrees off of exact 45 was met by a huge turn toward the corners. That is 0.5 deg *perpendicular* to the line cusp. They don't bounce backwards like you see in point cusps, which is what I expected, they just turn.

[edit: honestly I don't remember now what the maximum angle was for bounce back. It was above 0.5 and no more then 1.5 degrees of of 45, but I can't remember exactly because I truncated the date above it so the max that I simulated is different.]

At a certain angle they follow the corner cusp as you can see, but larger angles then that are funny because they turn so much that they "hit" the line cusp of an adjacent face. That line cusp is perpendicular to the one it was "following", and then causes the bounce back into the device. My interpretation is that in conical cusps the line cusp is effectively infinite which means the electron just turns until it escapes. But in polywell the line cusp is finite, so many angles which are normaly loss vectors are not because the line cusp is finite. Just guessing the more segmented you make the line cusps I imagine the more pronounced that becomes. I don't know that from experience though, just a guess.

I never though of using an image coil but it's ingeniously simple. I may try to implement it. I have been working on a completely different simulation method but this seems rather simple just to try.

here is that image I refered to. the initial angle between each shot is equal distant:

[rnebel]

Just a couple of points of clarification:

1. There were some questions about how one knows that you have "wiffleball" confinement as opposed to "mirrorlike" confinement. The answer is that there are about 3 orders of magnitude difference in the confinement times between the two modes. That kind of difference is easy to see.

2. The issue of the line cusps (or "funny cusps" as Dr. Bussard called them) is an interesting one. However, one thing that needs to be remembered is that electrons recirculate through the cusps and the confinement is electrostatic as well as magnetic. What matters is how often the electrons hit the coil casings.

[Art Carlson]

The latest picture from Carter is wierd. I don't understand it at all, especially since he seems to be using square coils, where I would expect such effects to be smaller. Is there a significant difference between the electron trajectories and the field lines? Can you give us a picture of the whole plane. (Half-plane is enough.) The field lines - but not the trajectories - should lie exactly in this plane. How about a reverse picture showing where the trajectories that hit the line cusp at one point but different angles come from. I don't want to make work for you, but I don't know what to make of this.

[kcdodd]

I wasn't totally sure what you want plotted. Here are the magnetic field lines of the simulation. The plotting tool only lets me do streamline of magnetic vectors, so I start all the lines in the faces of the coils.

In this one there are two vertical sets of start points on the x=1.5 face, at y=0.0 [red] and y=0.1 [blue], both going from 0.1 <= z <= 0.7.
http://www.andromedaspace.com/files/magfield1.png

same from different angle.
http://www.andromedaspace.com/files/magfield2.png

This one also has two sets on the x=1.5 face, but at z=0.1 [red] and z=0.2 [blue], both going from 0.0 <= y <= 0.2.
http://www.andromedaspace.com/files/magfield3.png

Same from different angle.
http://www.andromedaspace.com/files/magfield4.png

Only the red plot, the closest one to the line cusp.
http://www.andromedaspace.com/files/magfield5.png

[tombo]

As I see it the escape mode for the 2 coil cusp device is:
An electron (or ion) moving along the axis moving toward the center orbits a certain number of flux lines.
Any orbits that contain the central flux line will contain lines that diverge.
As it approaches the center the flux lines encircled diverge and eventually exit on the equator all the way around.
Since the number of flux lines contained in its orbit is conserved its orbit takes it out of the device.
Is that right?

[aside: Has anyone tried a 2 coil cusp machine with recirculation? It just might work. I think this was mentioned in another thread too.]

If we look at a Polywell the same way, where does the electron go?

The electron approaches the center along an axis circling a bundle of flux lines that that includes the central flux line and go to different places.
It spirals toward the center orbiting larger and larger radii then it (the guiding center) reverses direction to spiral away from the center again.
(After that reversal it contains more and more flux lines but half of the new ones go the other way also, so they cancel out.)
The encircled flux lines split up and go out of the device through 4 different corner cusps and the 4 connecting line cusps.
So this orbit takes it around the coil circling the major axis passing through all 4 corner cusps and all 4 line cusps following a square-ish path before it becomes more circular again outside the magrid.
Does the non-circularity of the loss path help? Or does it just wiggle its way around through them all?
I think it wiggles around them all and takes a square-ish path as it passes out between the coils following the line cusps.
[I think this is not a problem for the octahedral designs because there will always be a coil in the way.]
[There will probably be a coil support in the way in the cube version.]

My proposal:
Now add 8 coils just outside of the corners lined up in the shadows of the 6 primary coils.

Like this:


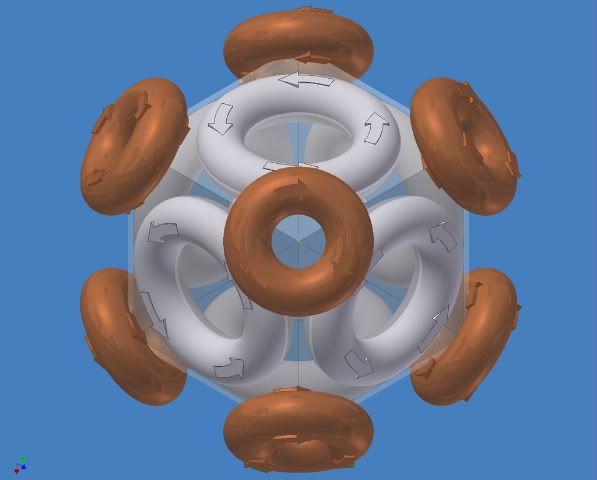
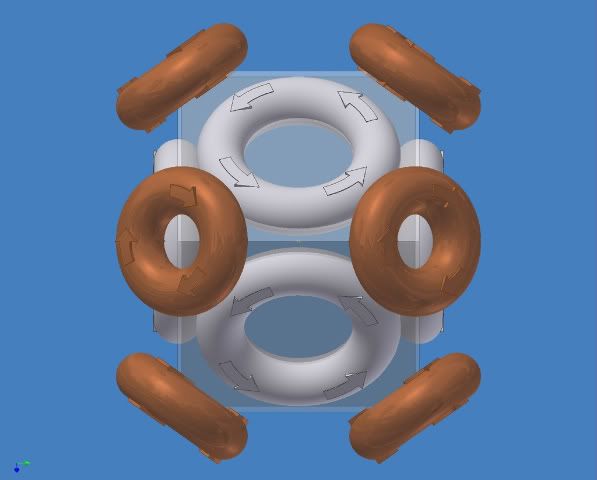
Most of the flux lines through the corner cusp will be gathered up by the outer coil and go through the outer coil.
This includes gathering up all of the central lines which form the tricuspid corner cusp and forces them to go through a point cusp.

Now no electron can escape without passing through a point type cusp.
(There will be a low field region between the outer coil and the virtual coils of the inner coils at that corner.
It is like between the turns of a solenoid. I don’t think it will be a problem but it will need to be analyzed.)
Now follow the electron trajectory of any electron whose orbit contains the central flux line.
It starts by spiraling inward but at some point it turns around and starts spiraling outward and passes through the magrid following a squarish orbit.

But now look what happens: four bundles of flux lines diverge to each go through a separate outer coil.
Now for the electron to continue follow this flux tube it must go out of and then back in through each of the 4 outer coils in turn.
The orbit becomes convoluted. But no matter how convoluted it becomes it will always be tied to the outer rings so it cannot get away. (except by collision etc.)

I consider this to be a work-around if the cusps don’t close enough when in wiffleball mode.

[kcdodd]

I have thought about that too. Every coil reinforces the point cusps of the adjacent coils. You can see though that the face coils all point out at the edges, and the corner coils all point in at the edges. They interfere each other on all of the line cusps, and you are left with very week fields there.

In the purest form this is just the polyhedral design. I extended it a bit and plotted with electric drive fields. In the simulation, however, the zero fields caused undefined behavior whenever electrons encountered them. Which lead me back to thinking the "standard" polywel was better. On top of that I have come to think that the asymmetric fields are actually a benefit to the design.

This plot shows only a single electron path trapped in the polyhedral device for 20 microseconds. The color bar gives the time in seconds. You can see it has very "weird" behavior.

http://www.andromedaspace.com/files/Hed ... 0k2_pc.png

http://www.andromedaspace.com/files/Hed ... 00k2_z.png